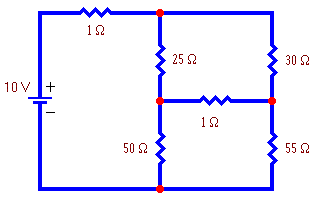
An electric network is a set of voltage sources and resistances connected in some way.
In this diagram we have removed all the resistors and voltage sources so that we can focus attention on the topology of the network (ie. the structure of the circuit) and count its nodes and branches.
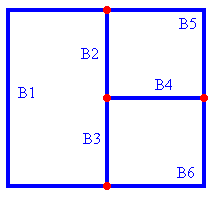
"To solve a network" means to find the current flowing in each branch of the network. Since this circuit has 6 branches, this means calculating 6 branch currents.
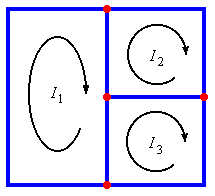
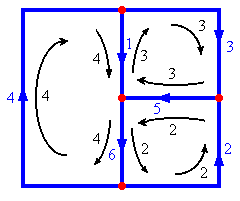
Loop currents offer a more economical way to describe the current flow in a network. The currents in all 6 branches can be described in terms of just 3 loop currents as shown to the right. A loop current is defined as a constant current that flows around a closed path or loop. (A closed path is a path through the network that ends where it starts.)
Each branch current is given by the algebraic sum of all the loop currents present in that branch. (By algebraic sum we mean that the sign and direction of loop currents must be taken into account in the sum.)
For example, if the 3 loop currents in the picture above have these values:
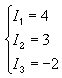
then the 6 branch currents in the picture to the right have the magnitudes and directions shown.
This drawing shows in detail how the various loop currents contribute to the various branch currents.
Here is a different set of 3 loop currents. If the values of the loop currents are:
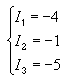then the 6 branch currents have exactly the same values as
before.
