BCIT ~ Mathematics - Examples - Nuclear Medicine

In nuclear medicine minute quantities of radioactive materials with short half lives such as Iodine - 131 (half-life 8.065 days) or Technetium - 99 (half-life 6.007 hours) can be injected into patients, the material traced and then a medical diagnosis made.,

Since the radioactive material is constantly decaying, the label on a bottle will state the radioactivity in the bottle at a given moment in time. Patient doses will need to be drawn up for a specific time that differs from that on the bottle.

The relevant formula is A = A0 2 - t / T, where

A0 = the initial radioactivity at time t = 0
A = the radioactivity at any later time t
T = the half-life (a known constant for a given radioisotope)

Note that t and T must have the same units. The metric unit for radioactivity is the Bequerel.

(1 Bq = 1 disintegration/second)

(1 MBq = 1 million disintegrations/second)

Question:

A bottle of Iodine-131 is delivered on Nov. 3rd from the manufacturer and it states on the label that the activity will be 370 MBq at 1200 hours on Nov. 11th. What is the activity

at 1200 hours on the delivery day?
at 0800 hours on the 27th of November?

Solution:

We know that:

The half-life of Iodine-131 is 8.065 days
From Nov. 3 to Nov. 11th is 8 days
From Nov. 11th 1200 h to Nov. 27th at 0800h is (16 days less 4 hours) =15.833 days

Let A0 denote the (unknown) initial acitivity on delivery day, Nov 3rd, at 1200 h. Since it is known that 8 days later the activity is 370 MBq:
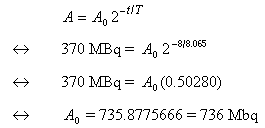
Answer: The activity on delivery day, Nov 3rd, at 1200 h, is 736 MBq.
For this part, consider the activity 370 MBq at 1200 hours on Nov. 11th to be the initial activity, A0. Let the unknown activity 15.833 days later (on Nov. 27th at 0800 h) be A:
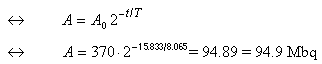
Answer: The activity on Nov. 27th at 0800 h, is 94.9 MBq.

Nuclear Medicine: Radioactive Half-Lifes and Exonentials

Question:

Solution: