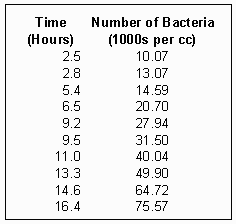
The experimental measurements listed above indicate the number
of bacteria are growing with time. If we plot the observed values
of N (vertically) against the elapsed time, t, in hours, we get
the graph:

You can draw a smooth curve through the experimental points as
shown in this figure, and the points all seem to lie relatively
close to this curve. Further, this curve looks quite a bit like
the graph of an exponential function, but from what we see, it
might just as well be part of a parabola or of one of a number of
other curves that result when various algebraic forms are
graphed. This graph suggests that the answer to the first question may be
"yes", but we would usually want stronger evidence than
this to draw a definite conclusion. The trouble here is that it
is difficult to distinguish precisely and reliably between the
curves are the graphs of quite different mathematical
functions.
There is, of course, one curve that is easily recognized by
everyone - the straight line! In this situation, the logarithmic
function can be used to rewrite the exponential growth formula
into a form that will allow us to create a plot that does give a
straight line when data is the result of an exponential growth
(or decay) process. Start with the exponential growth
formula:
N = N0 ekt
Since this involves an exponential with base e, it is
"natural" to use natural logarithms - those based on
the number e. Using the more common notation ‘ln’
for ‘loge’, we simply take the
logarithm of both sides of the previous equation:
ln N = ln(N0 ekt)
= ln N0 + ln(ekt)
= ln N0 + kt ln e
= ln N0 + kt
Here, we have used two of the three basic properties of
logarithms to manipulate the right-hand side, namely that
log (AB) = log A + log B
log (Am) = m log A
Further, in the last step we took advantage of the fact that
by choosing to work with natural logarithms, ln e =
logee = 1. Thus, the exponential growth formula can be
written in the logarithmic form
ln N = kt + ln N0
If the exponential growth formula is correct and we were to
plot ln N along the vertical axis of our graph (rather than N
itself), and t along the horizontal axis, then the equation of
the plot we are preparing will have the generic form
vertical quantity = constant x horizontal quantity + another
constant
But this is just the equation of a straight line. The plot
that results using the data given above is:
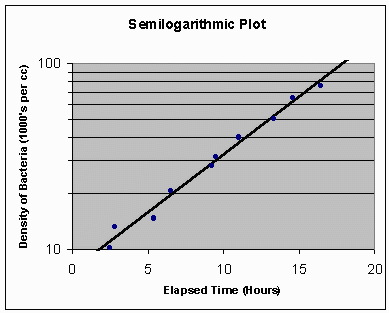
Although the values written on the vertical axis are values of
N, this graph is ruled vertically so that the vertical distances
are actually proportional to ln N. Since one scale is logarithmic
(the vertical one) whereas the other graph scale, the horizontal
one, is the ordinary uniform spacing type, this is called a
‘semilogarithmic plot’.
At any rate, the figure indicates that the points are
scattered about a straight line, the scatter due to small random
errors in measurement. Since the plot of ln N vs t gives a
straight line, we have confirmed that
ln N = kt + ln N0 (equation *)
is a valid formula for this data, and therefore, so is its
equivalent, N = N0 ekt, valid for this data. This ends our
answer to the first question posed.
It is now easy to answer the second question posed. Since we
have concluded that (*) correctly describes the straight line
graph in the semilogarithmic plot, we notice that the constant k
is just the slope of this line. To calculate the slope, we first
read the coordinates of two points on the line. Working
with some care, for instance, we can determine that two such
points are:
t = 1.80, N = 10
t = 17.95, N = 100
(for this sort of work, it’s important to pick your
points as widely spaced as possible). These give us
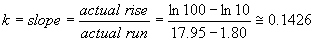
Notice that the ‘actual rise’ has to be the
actual vertical distance between the two points, and because the
vertical scale is ruled off in proportion to ln N, this vertical
distance will be the difference between the natural logarithms of
the N values for the two points.
It would be easy to read the value of N0 directly
off of the graph if we could see the point where the straight
line crosses the t = 0 position. This is because when t = 0, we
of course have simply
N = N0
and so the value of N0 could be read directly off
of the graph. Unfortunately, because of the way we cropped our
semilogarithmic plot, this is not possible in this example.
Instead, we can substitute the coordinates of one of the points
we’ve read from the graph into formula (*) above to
get
ln 100 = (0.1426)(17.95) + ln N0
to give
ln N0 = ln 100 - (0.1426)(17.95) ≅ 2.04595
Thus,
N0 ≅ e2.04595 ≅ 7.737.
Finally, to compute the doubling time, t2, for this
system, the time required for the number of bacteria to double,
we use the formula

(The units on t2 come about because if you trace
the work above carefully, you find that k has units of
hours-1. Also, you can derive
the formula above from the original formula describing
exponential growth by substituting N = 2N0 and solving
for t.)
In summary then, we have obtained the following results:
- we have confirmed that the data given at the beginning of
the problem is indeed consistent with an exponential growth
model.
- based on that we have been able to estimate the constants
in the exponential growth formula for this system, to get
N ≅ 7.737 e0.1426t
- based on these results, we have determined that the number
of bacteria will double approximately every 4.86 hours.